Targeting Nominal GDP Through Monetary Control

The statutory dual mandate of the Federal Reserve (Fed) instructs the US central bank to conduct monetary policy “so as to promote effectively the goals of maximum employment, stable prices, and moderate long-term interest rates.”¹ Congress leaves it to the Fed to choose an operational strategy that best achieves these goals.

The Fed presently uses an operational strategy called flexible average inflation targeting (FAIT). This policy brief proposes that at the Fed’s next major review, planned for 2024–25, it should replace FAIT with an alternative operational strategy that targets nominal GDP (NGDP) instead. More specifically, the Fed should target NGDP by influencing broad measures of the money supply such as M2 or controlling the monetary base directly. This approach would allow the Fed to pursue its dual mandate of maxi mum employment and economic stability whether or not interest rates are constrained by their zero lower bound (ZLB).

This brief retraces the steps that led the Fed to adopt its current framework—that is, FAIT. It then describes how the alternative strategy of NGDP targeting via monetary control addresses more effectively the problems that FAIT was intended to solve.

Many economists, both inside and outside the Fed, believe that the links between money and NGDP growth have weakened in recent decades. To counter this impression, the brief demonstrates that, once slow-moving trends in monetary velocity are accounted for, fluctuations in the broad monetary aggregates and the monetary base are still followed reliably by movements in NGDP. These statistical links can help the Fed implement a new NGDP targeting strategy.

The same statistical links can be used by outside observers to interpret and predict the effects of monetary policy—even if, following the upcoming strategic review, the Fed decides to stick with some variant of FAIT.

The Path to FAIT

During the 1990s, flexible inflation targeting emerged as the consensus best practice among central bankers around the world.² As its name indicates, the inflation targeting strategy specifies a quantitative target for inflation that the central bank aims to achieve, typically on an annual basis. But the strategy retains a degree of flexibility that allows policymakers to pursue stabilization goals for employment as well.

Flexible inflation targeting thereby remains consistent with the Fed’s dual mandate. In fact, the Federal Open Market Committee (FOMC) explicitly adopted this strategy in January 2012. The statement outlining the strategy begins by explaining that the FOMC “judges that inflation at the rate of 2 percent . . . is most consistent with the Federal Reserve’s statutory mandate,” but goes on to note that in “setting monetary policy, the Committee seeks to mitigate deviations of inflation from its longer-run goal and deviations of employment from the Committee’s assessment of its maximum level.”³

Historically, the FOMC has implemented flexible inflation targeting by managing the federal funds rate, raising or lowering the funds rate when inflation or employment or both rise above or fall below desired levels. Throughout the severe recession of 2008–09 and the slow recovery that followed, however, the FOMC was stymied in this approach by the zero lower interest rate bound. Even after lowering its federal funds rate target to a range near zero in late 2008 and holding it there through 2015, the FOMC could not bring inflation all the way back to 2 percent.

The FOMC addressed the problem of the ZLB during its 2019 strategic review by adopting a modified version of flexible inflation targeting it called “flexible average inflation targeting.”⁴ The 2020 amendment to the FOMC’s strategy statement describes this change, noting that “the federal funds rate is likely to be constrained more frequently than in the past.” Therefore, “the Committee seeks to achieve inflation that averages 2 percent over time, and . . . judges that, following periods when inflation has been running persistently below 2 percent, appropriate monetary policy will likely aim to achieve inflation moderately above 2 percent for some time.”

Unfortunately, under FAIT, inflation has risen sharply—not just “moderately”—above 2 percent.⁵ Thus, the question that will confront the FOMC in its next strategic review seems clear: How can the Fed pursue its dual mandate more reliably while still accounting for the constraint imposed by the ZLB? Mercatus Center economists David Beckworth and Patrick Horan provide one answer to this question by identifying ways to eliminate the problems that have prevented FAIT from being as effective as originally hoped.⁶ As noted earlier, this policy brief presents an alternative: nominal GDP targeting, achieved through the Fed’s ability to influence broad measures of the money supply such as M2 or to control the monetary base directly.⁷

NGDP Targeting and the Money Stock

NGDP targeting, also known as nominal income targeting, is often proposed as an alternative to flexible inflation targeting as a strategy for pursuing the dual mandate. Cato Institute scholar George Selgin surveys the literature that advocates NGDP targeting, a literature that extends back into the 19th century.⁸ University of Mississippi economics professor Joshua Hendrickson shows how, during the 1980s and 1990s, the Fed stabilized NGDP and thereby helped create the era of low inflation and robust economic growth known as the Great Moderation.⁹ Mercatus Center scholar Scott Sumner, meanwhile, argues that the Federal Reserve’s failure to stabilize NGDP lies behind the severity and length of the Great Recession of 2008–09.¹⁰ David Beckworth enumerates the many advantages of targeting NGDP in levels rather than growth rates, and Haverford College economics professor Carola Binder describes how monetary policy based on NGDP targeting becomes easier for the public to understand.¹¹

Some—but by no means all—of the arguments favoring NGDP targeting run as follows. First, as a nominal variable measured in dollars, NGDP is under the clear influence of the central bank: Through appropriate monetary policy actions, over periods of several years the Fed can achieve any average growth rate of NGDP. At the same time, since NGDP is the product of the nominal price level and real GDP, policy actions that stabilize NGDP growth also stabilize an equally weighted average of inflation and real GDP growth. Hence the strategy is, by design, consistent with both sides of the Fed’s dual mandate. Yet the strategy avoids any reference to the famous (or infamous) Phillips curve relationship between inflation and unemployment that often exhibits instability, leading Fed policy astray.¹² Finally, stabilizing NGDP helps the Fed respond appropriately to different types of macroeconomic shocks by “leaning against the wind” in response to aggregate demand disturbances that move prices and output in the same direction while also “seeing through” the effects of aggregate supply shocks that move prices and output in opposite directions.¹³

Simply asking the Fed to stabilize NGDP won’t suffice, however. Though nominal income is clearly under the influence of the central bank, it is not a variable that the Fed can control with high precision on a quarterly or perhaps even an annual basis. A complete description of an operational NGDP targeting strategy must also specify exactly how the FOMC intends to achieve the desired stability in NGDP.

To this end, David Beckworth, Joshua Hendrickson, and MIT economics professor Athanasios Orphanides propose monetary policy rules that target NGDP through the FOMC’s standard practice of managing the federal funds rate.¹⁴ These rules have the advantage of familiarity: They are variants of the famous Taylor rule, which is used to describe how, historically, the Fed has managed the funds rate to implement flexible inflation targeting.¹⁵ These rules, however, fall victim to the ZLB: They don’t prescribe what the FOMC should do during a deflationary recession once the federal funds rate target hits zero. Another operational strategy has been proposed by Scott Sumner: to implement NGDP targeting by stabilizing the price of a publicly traded futures contract based on NGDP.¹⁶ Sumner’s unique brand of market monetarism works both at and away from the ZLB, but it would require major innovations in the way the Fed intervenes in financial markets.

In between these two extremes—the traditional approach of targeting the funds rate and the highly innovative approach of targeting futures prices—economists Bennett McCallum, Martin Feldstein, and James Stock describe how the Fed could stabilize NGDP by controlling the monetary base or influencing the broader monetary aggregates such as M2.¹⁷ These proposals, however, were made during the late 1980s and early 1990s, when economists, inside and outside the Fed, monitored the monetary base and M2 much more closely than they do today.

More recently, large and persistent movements in monetary velocity have weakened the direct statistical links between these measures of the money supply and NGDP. To cite the most glaring example: The monetary base expanded dramatically, from less than $900 billion in the third quarter of 2007 to more than $4 trillion in the third quarter of 2014, without kindling a noticeable acceleration in NGDP growth.¹⁸ Fluctuations in M2 velocity have been less extreme but still large enough to disturb the links between M2 and NGDP growth, documented by Feldstein and Stock. In congressional testimony, Federal Reserve Chair Jerome Powell summarized well the beliefs of most central bankers and economists in 2021:

Well, when you and I studied economics a million years ago, M2 and monetary aggregates seemed to have a relationship to economic growth. Right now, I would say the growth of M2 . . . does not really have important implications for the economic outlook. . . . We have had big growth of monetary aggregates at various times without inflation, so [it’s] something we have to unlearn, I guess.¹⁹

As the next sections show, however, a standard quantity-theoretic model, called the “P-star” model, can account for these changes in velocity, allowing statistical analysis to recover much stronger links between measures of the money stock and NGDP, even in the most recent data. Tests of this model confirm that a strategy of NGDP targeting can be implemented through policies of monetary control, thereby sidestepping the problem of the ZLB.

The P-Star Model of Money and Nominal GDP

Federal Reserve economists Jeffrey Hallman, Richard Porter, and David Small developed the P-star model in the late 1980s at the request of then-chairman Alan Greenspan.²⁰ By linking the money stock to the price level, the model was designed to help the Fed implement a flexible inflation targeting strategy. It is based on the equation of exchange,

M_tV_t = P_tY_t ,     (1) 

where M_t denotes a measure of the money stock at time t, V_t is the corresponding measure of velocity, P_t is the aggregate price level, and Y_t is real GDP.

Equation (1) holds as an identity by virtue of the definition of velocity as NGDP divided by the money stock. The P-star model gives this equation testable implications and predictive power by making assumptions about the behavior of velocity and real GDP. To accomplish this, Hallman, Porter, and Small rewrite equation (1) to define the variable that gives their model its name, “P-star”:

In equation (2), V_t^* and Y_t^* denote the “natural,” “equilibrium,” or “trend” levels to which velocity and real GDP are expected to return in the long run. Both can vary over time, V_t^* because of persistent shifts in the demand for money relative to other assets and Y_t^* because of technological changes that generate long-run economic growth. The variable P_t^* then should be interpreted as the level to which aggregate prices will converge, given the current level of the money stock, as velocity and real GDP return to their own long-run levels.

In a manner consistent with the quantity theory of money, therefore, the P-star model allows increases in the money supply to be held temporarily as excess cash balances, thereby lowering velocity, or to temporarily stimulate spending, thereby increasing real GDP in the short run. The model implies, however, that as these effects wear off in the long run, any change in the money stock will be matched by a proportional change in the aggregate price level.²¹ Hallman, Porter, and Small test the P-star model with a regression equation:

 ∆π_t = α + β1∆π_t–1 + β2∆π_t–2 + β3∆π_t–3 + β4∆π_t–4 + γ(p^*_t–1 – p_t–1) + ε_t, (3)

where _πt equals 400[ln(P_t) – ln(P_t–1)] and denotes the quarterly inflation rate, expressed in annualized percentage-point terms; _∆πt equals _πt – _πt–1 and denotes the corresponding change in inflation; the lagged “price gap” variable p^*_t–1 – p_t–1 equals 100[ln(P^*_t–1) – ln(P_t–1)] and represents the percentage-point deviation of the equilibrium price level from the actual price level; and the regression error _εt is assumed to be uncorrelated with its own lagged values as well as with the other variables on the right-hand side of equation (3).

In equation (3), a positive and statistically significant estimate of the coefficient _γ confirms the model’s quantity-theoretic implication that inflation will accelerate when the price gap is positive, as P_t rises to meet P^*_t. Likewise, inflation will decelerate when the price gap is negative. The past changes in inflation included on the right-hand side of equation (3) allow the convergence of P_t to P^*_t to take place smoothly and with a longer lag. A positive and statistically significant estimate of _γ, therefore, implies that the P-star price gap is a useful indicator of the effects that past money growth will have on future inflation.

In recent research, University of Mississippi economics professor Michael Belongia and I modify the P-star model to apply to NGDP instead of inflation targeting and to account for the larger movements in velocity seen in US data since the early 1990s.²² We start by rewriting equation  (1) as

     M_tV_t = Q_t,     (4)

replacing the aggregate price level and real GDP on the right-hand side by their product: Q_t = P_tY_t. This product is, of course, NGDP. Next, we modify equation (2) to define the new variable “Q-star”:

     Q^*_t = M_tV^*_t .     (5)

In equation (5), V^*_t is defined, once again, as the equilibrium level of velocity. Therefore, Q^*_t is the equilibrium level of NGDP implied by the current level of the money stock, to which NGDP should converge as velocity returns to its own long-run level.

Comparing equations (2) and (5) reveals one key advantage of NGDP targeting over inflation targeting when it is implemented with reference to the P-star model: NGDP targeting via equation (5) does not require an estimate of the natural rate of output, Y^*_t.²³ On the other hand, equation (5) still requires an estimate of velocity’s trend value, V^*_t. Hallman, Porter, and Small in their original study selected M2 as their measure of money and took V^*_t to be constant, since M2 velocity fluctuated around a constant long-run value in quarterly US data from 1955 through 1988. Unfortunately, M2 velocity moved abruptly higher shortly after the publication of their article, throwing off the model’s predictions and prompting the more general concerns, noted earlier, about the usefulness of money in forecasting inflation.²⁴

Belongia and I show, however, that movements in equilibrium velocity are tracked closely by estimates provided by a “one-sided” version of the time series filter developed by economists Robert Hodrick and Edward Prescott to decompose economic variables into trend and cyclical components.²⁵ Essentially, the one-sided Hodrick-Prescott filter uses a long moving average of past values of velocity itself to compute a time-varying estimate of V^*_t. Importantly, the use of past data alone means that the model’s estimates of V^*_t and Q^*_t can be updated with information available to policymakers as they make decisions in real time.

Figure 1 plots the velocities of three broad monetary aggregates used in testing the P-star model. Simple-sum M2 is the Fed’s official measure, computed as the dollar value of funds held by the public in the form of currency, checking account balances, and savings account balances (these include money market deposit account balances, retail money market mutual fund shares, and small certificates of deposit). Divisia M2 includes the same assets as the Fed’s measure but reweights each component according to its degree of “moneyness”: Currency receives the highest weight and is higher-yielding but less liquid assets receive smaller weights.²⁶ Divisia M4 is an even broader weighted monetary aggregate that includes all the assets from M2 plus large time deposits, institutional money market mutual fund shares, overnight and term repurchase agreements, commercial paper, and US Treasury bills.²⁷ This aggregate has had especially high predictive power for economic activity and inflation in recent years, reflecting the increased importance of nonbank financial intermediaries—“shadow banks”—within the US economy.²⁸

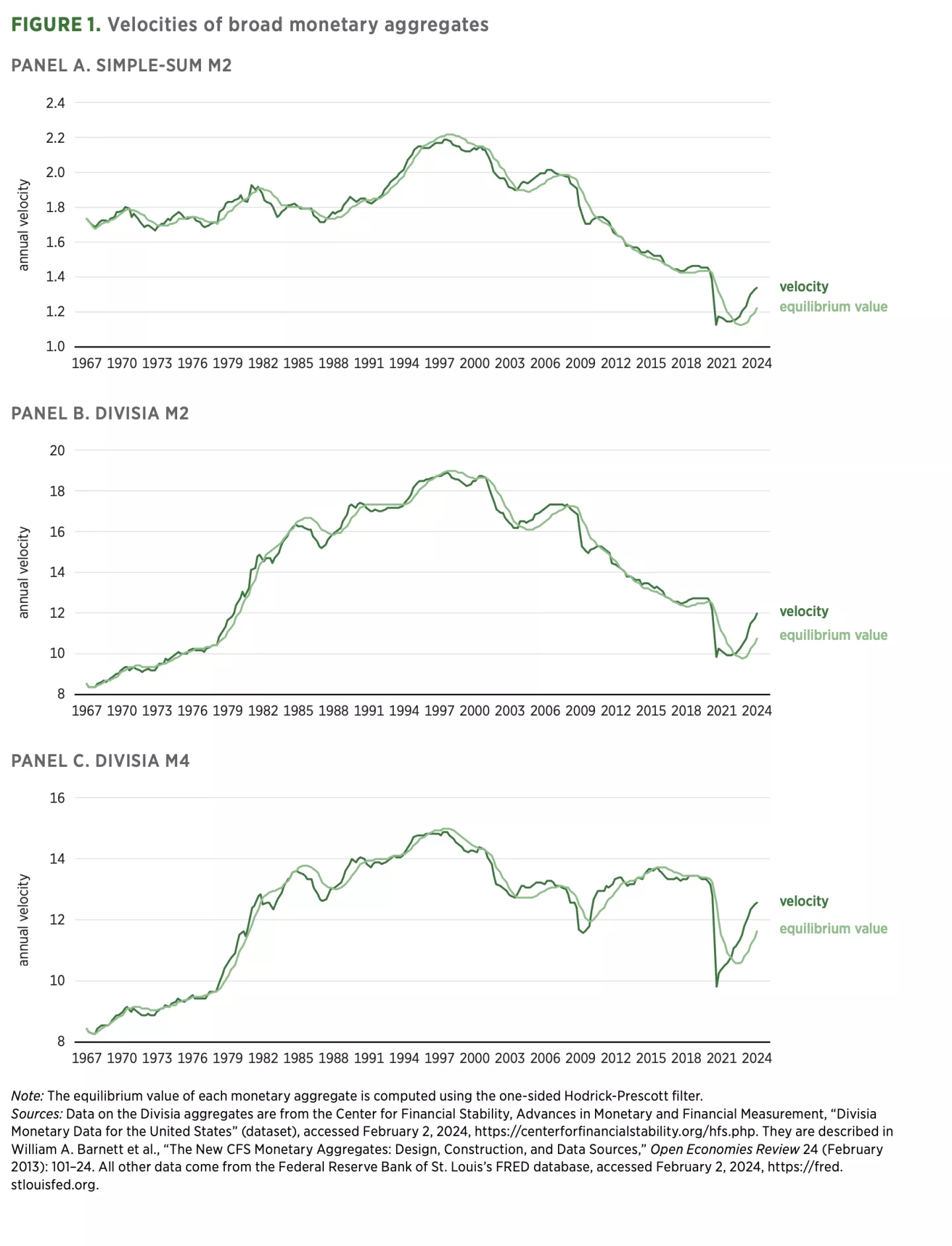
These broad monetary aggregates, consisting mainly of assets issued by banks and nonbank financial institutions, can be influenced but not directly controlled by the Federal Reserve. By contrast, the monetary base—consisting only of currency and bank reserves—is under the Fed’s strict control. Figure 2 plots the velocities of two measures of the monetary base.

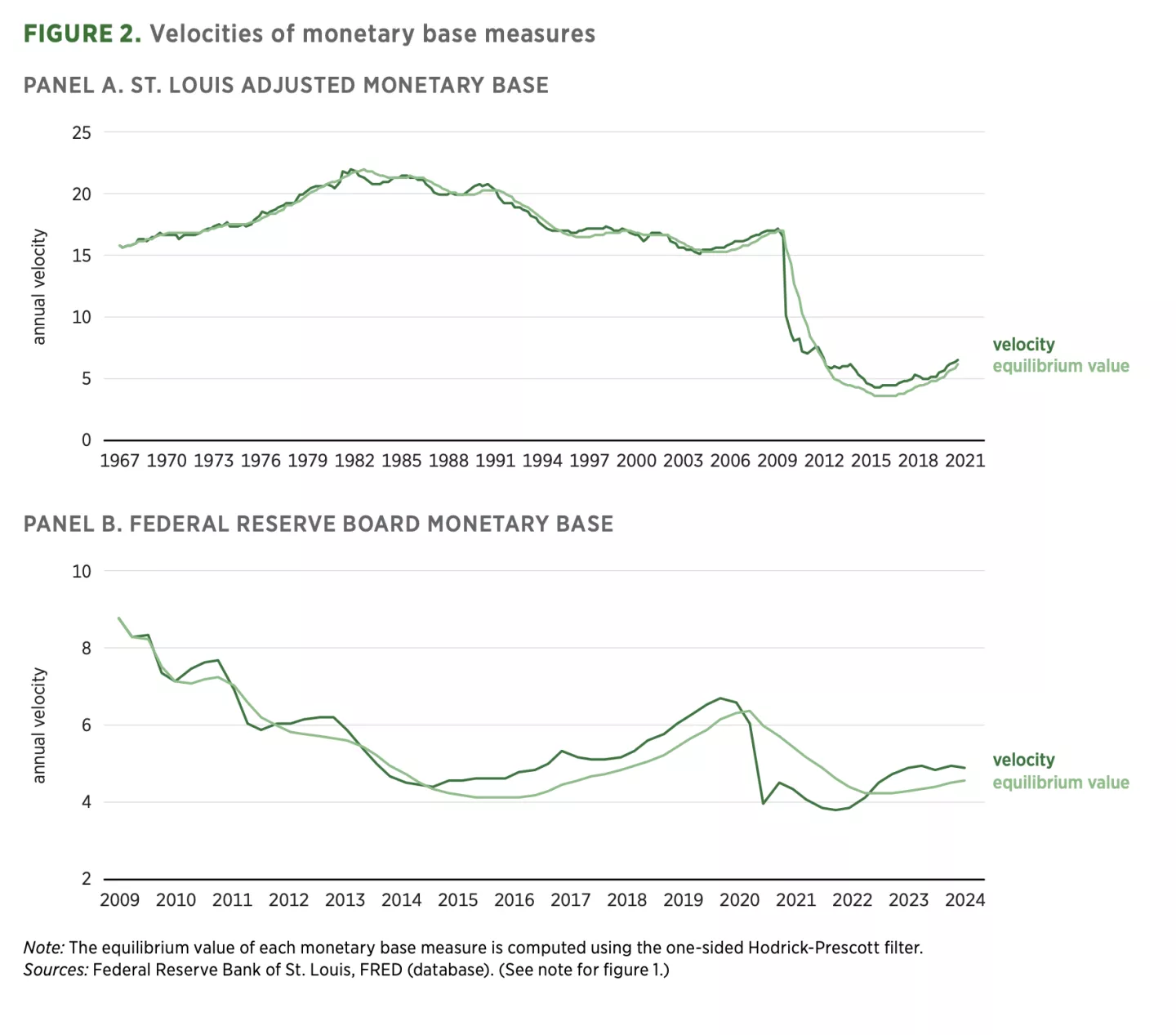
As its name indicates, the St. Louis adjusted monetary base is constructed at the Federal Reserve Bank of St. Louis and adjusts for changes in reserve requirements as well as for the “retail deposit sweep programs” banks used, starting in the mid-1990s, to minimize their required reserves.²⁹ Unfortunately, the St. Louis Fed discontinued this series in the fourth quarter of 2019. Hence, figure 2 also plots the velocity of the monetary base as computed by the Federal Reserve Board over the period since 2009. Unlike the St. Louis measure, the board’s base series is not adjusted for changes in reserve requirements, nor is it seasonally adjusted. Panel B reveals, however, that base velocity computed with the board’s measure since 2009 does not appear to contain important seasonal fluctuations.

Each panel of figures 1 and 2 compares velocity V_t to the corresponding estimate of V^*_t obtained from the one-sided Hodrick-Prescott filter. In every case—including, impressively, the St. Louis base series, which shows a massive decline in velocity in 2008—movements in V^*_t adapt quickly to changes in velocity itself, raising hopes that, after accounting for movements in trend velocity, the P-star model will remain useful for targeting NGDP in practice.

To test this hypothesis, the original P-star regression equation (3) is replaced here by

 ∆g_t = α + β₁∆g_t–1 + β₂∆g_t–2 + β₃∆g_t–3 + β₄∆g_t–4 + γ(q^*_t–1 – q_t–1) + ε_t, (6) 

where g_t equals 400[ln(Q_t) – ln(Q_t–1)] and denotes the quarterly growth rate of NGDP in annualized percentage-point terms; _∆g_t equals g_t – g_t–1 and is the change in NGDP growth; and the lagged “nominal GDP gap” q^*_t–1 – q_t–1 equals 100[ln(Q^*_t–1) – ln(Q_t–1)] and is computed as the percentage-point deviation between the equilibrium and actual levels of NGDP.

Just as before, a positive and statistically significant estimate of the coefficient _γ from equation (6) implies that NGDP growth will accelerate when the nominal GDP gap is positive, as Q_t rises to meet Q^*_t. Likewise, NGDP growth will decelerate when the nominal GDP gap is negative. And, as before, the lagged changes in NGDP growth included on the right-hand side of equation (6) allow this convergence of Q_t to Q^*_t to take place smoothly with a lag.

Thus, a positive and statistically significant estimate of _γ from equation (6) implies that the Fed could use its influence over a broad monetary aggregate or its direct control over the monetary base to successfully implement an NGDP targeting strategy through monetary control. In particular, the Fed could indirectly stimulate broad money growth or directly increase the monetary base to increase Q^*_t via equation (5) and thereby put upward pressure on NGDP growth. Likewise, it could act to reduce Q^*_t and put downward pressure on NGDP growth.

Results and Conclusions

Table 1 displays results when equation (6) is estimated with quarterly data on the broad monetary aggregates from a long sample running from quarter 1 of 1967 through quarter 4 of 2023.³⁰ The estimates of the key parameter _γ are large, associating a one-percentage-point nominal GDP gap with an acceleration in NGDP growth, one quarter later, ranging from 0.57 to 0.65 percentage points. These estimates are highly significant as well: p values less than 0.01 reject the null hypothesis that the coefficient equals zero with an extremely high degree of confidence.

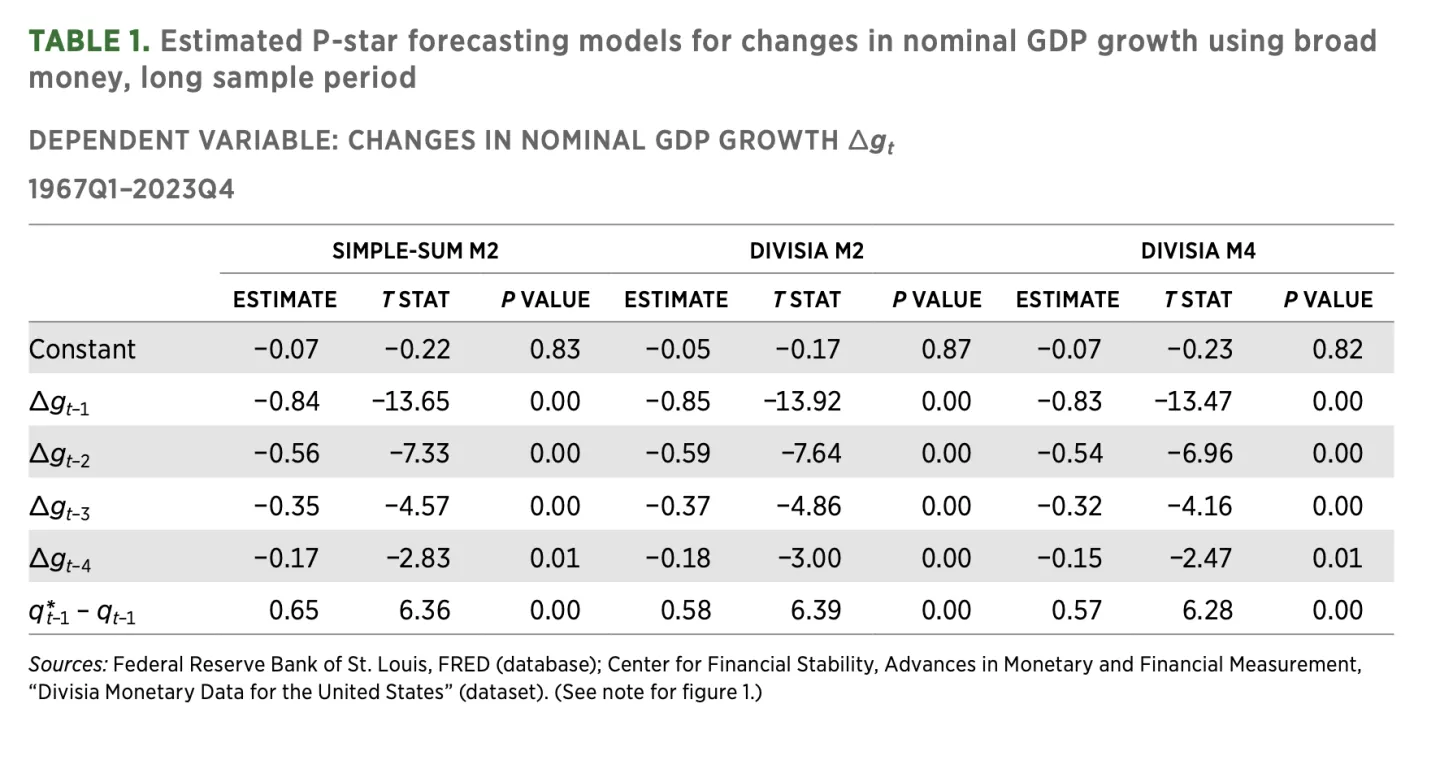
Since, as noted above, the predictive power of broad money growth is widely believed to have weakened in recent decades, table 2 examines two subperiods: before and after 1980. Indeed, estimates of _γ decline from around 0.90 before 1980 to 0.60 after. But even the post-1980 estimates associate the lagged nominal GDP gap with a sizable acceleration in NGDP growth. And estimates from both subsamples remain highly significant.

Table 3 zooms in on two recent periods: the first starting in 1980 and running through 2007 and the second covering the period since 2008, when the Fed has been constrained repeatedly by the ZLB. The estimates of _γ across these two subsamples show that the effects of broad money growth on NGDP have actually become stronger since 2008. None of these results appears sensitive to the choice between monetary aggregates: simple-sum M2, Divisia M2, or Divisia M4.

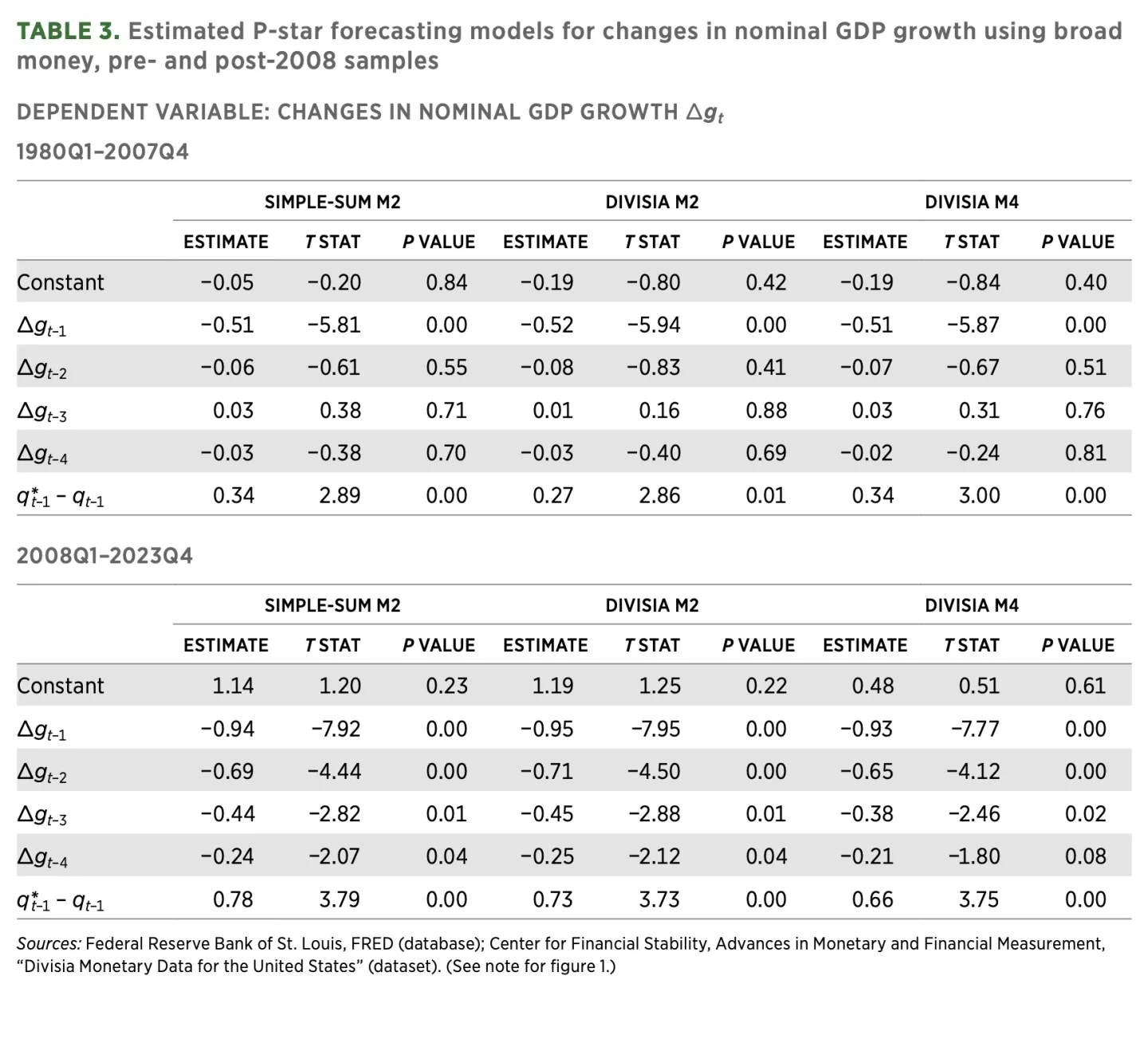
Table 4 focuses on estimates of equation (6) using the two measures of the monetary base. As noted earlier, base velocity declined sharply in 2008, when the Fed began paying interest on bank reserves. Not surprisingly, therefore, the parameter value _γ = 0.04 appears small when estimated with quarterly data on the St. Louis adjusted monetary base running from quarter 1 of 1967 through quarter 3 of 2019.³¹ But even in this case, the p value for testing the null hypothesis that this key coefficient equals zero falls below 0.10, which means the hypothesis can be rejected with 90 percent confidence.

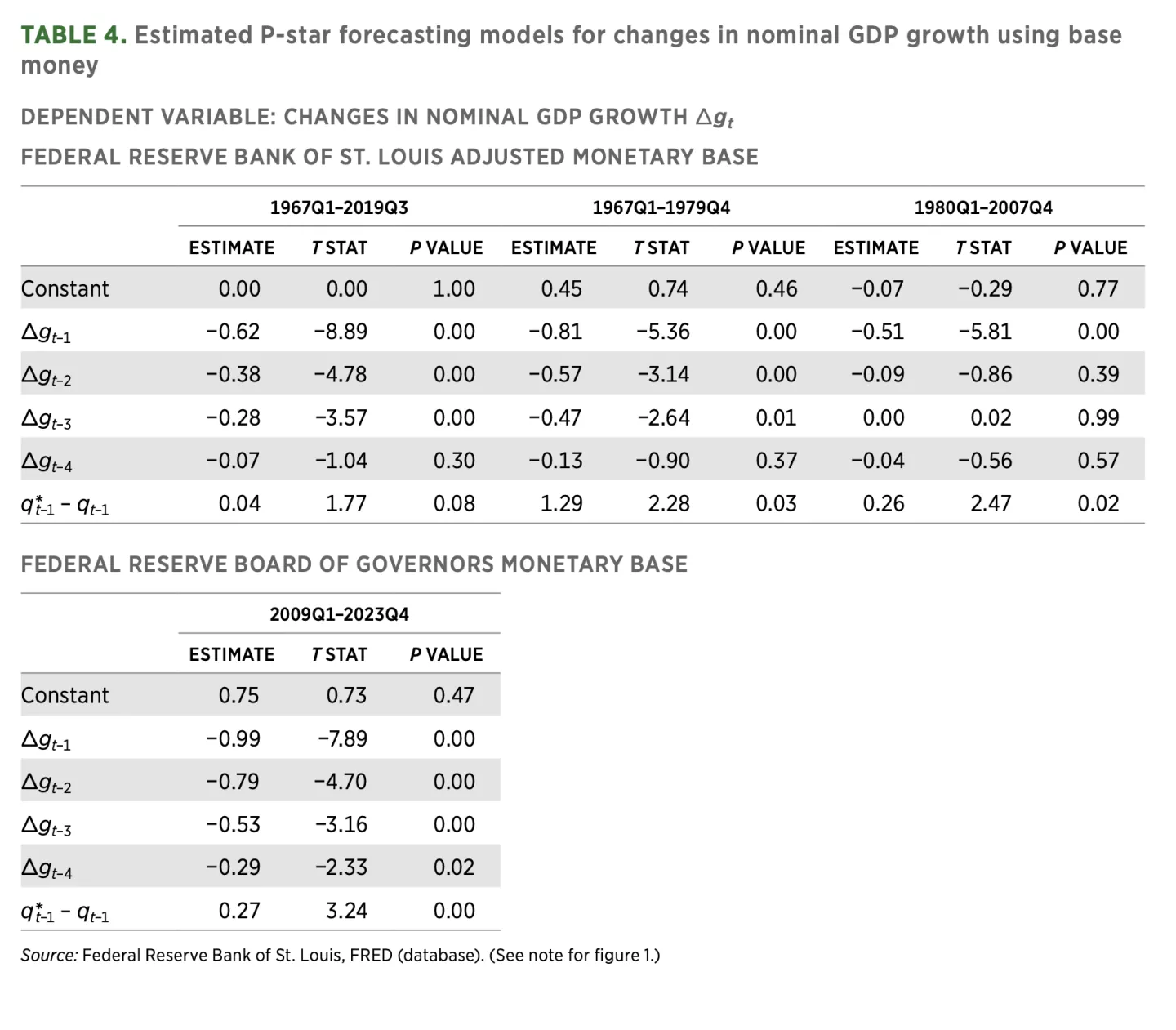
Stronger results reemerge when equation (6) is estimated with data over separate subsamples, using the St. Louis base measure for periods running from quarter 1 of 1967 through quarter 4 of 1979 and from quarter 1 of 1980 through quarter 4 of 2007 and using the Federal Reserve Board’s measure for a period running from quarter 1 of 2009 through quarter 4 of 2023—thereby allowing for a one-year transition in 2008 during which banks adjusted their demand for reserves in response to the Fed’s decision to pay interest on reserves. Once more, the estimated value of γ falls after 1980 but remains sizable in both recent periods, associating a one-percentage-point increase in the nominal GDP gap with a quarter-percentage-point acceleration in NGDP growth one quarter later. And in each of the three subsamples, the estimate of _γ regains a high degree of statistical significance.

These results confirm that, once slow-moving trends in velocity are accounted for, changes in both the broad monetary aggregates and the monetary base have strong predictive power for future NGDP growth. These results imply that the Fed could use its ability to influence broad money growth, or its direct control over the monetary base, to successfully implement a strategy of NGDP targeting. And by refocusing the FOMC’s attention on money growth instead of interest rates, this approach would allow the committee to pursue and communicate an NGDP targeting strategy consistently, both at and away from the ZLB. The results thereby support the idea that NGDP targeting through monetary control represents a preferable alternative to the flexible average inflation targeting strategy that the Fed has been using since 2020.

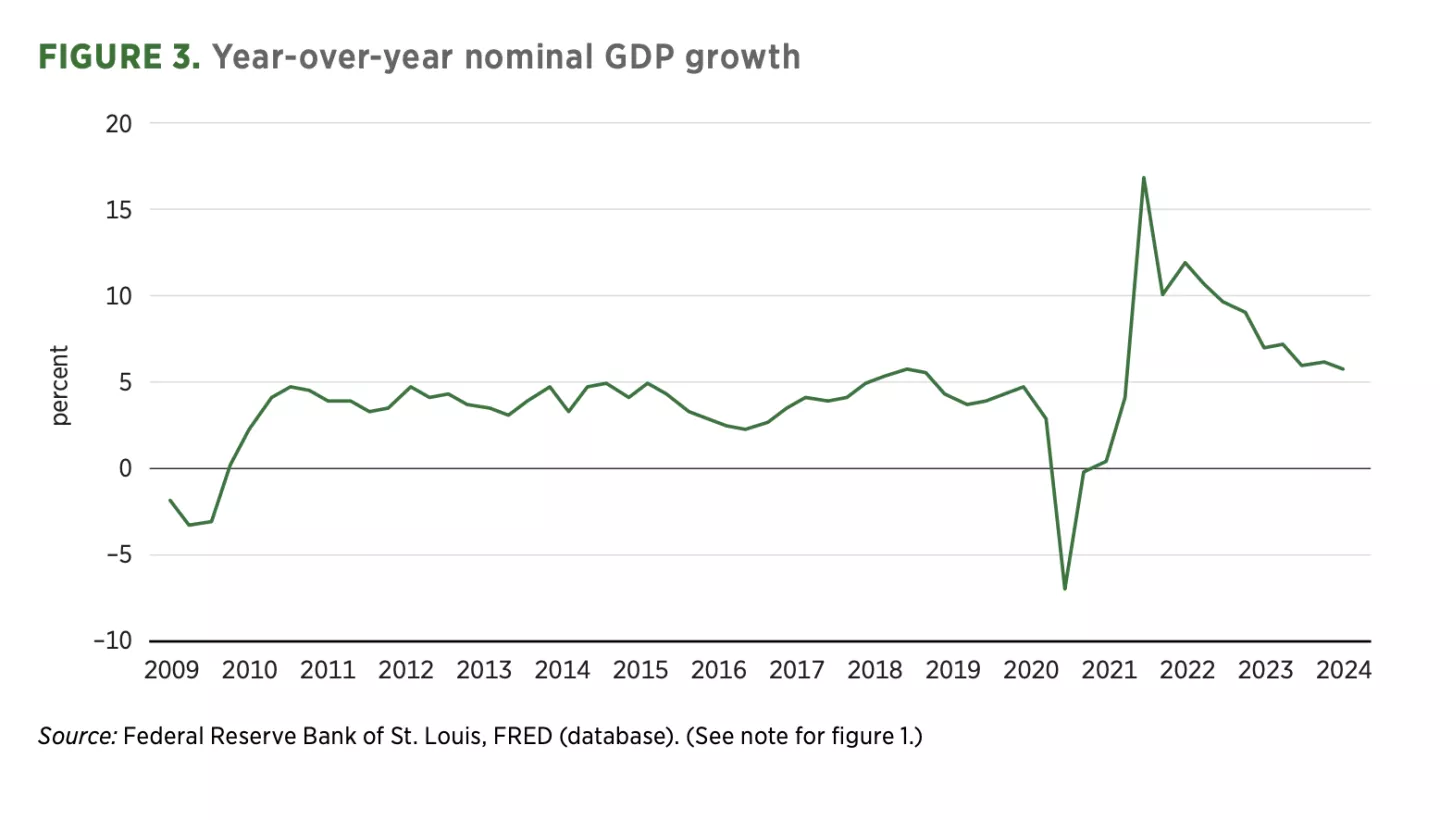
In addition to providing guidance to the FOMC as it looks forward to its upcoming strategic review, the results presented here can be used by outside observers who wish to measure and predict the effects that the Fed’s monetary policy actions will have on the economy. In fact, the approach outlined here can be used by Fed watchers even if the FOMC chooses to continue to manage the federal funds rate to implement some version of FAIT. To illustrate how, figure 3 plots year-over-year growth rates of NGDP since 2009. The graph shows the extended period of moderate and stable NGDP growth extending from 2011 through 2019, the sharp decline in nominal spending during the 2020 economic closures, and the even more dramatic acceleration in NGDP growth reflecting the unwanted rise in inflation since 2021.

More recently, NGDP growth has been trending downward. But will this trend continue? To help answer this question, figure 4 plots the four measures of the NGDP gap—based on simple-sum M2, Divisia M2, Divisia M4, and the Federal Reserve Board’s measure of the monetary base—used in estimating equation (6) over periods running through the present.

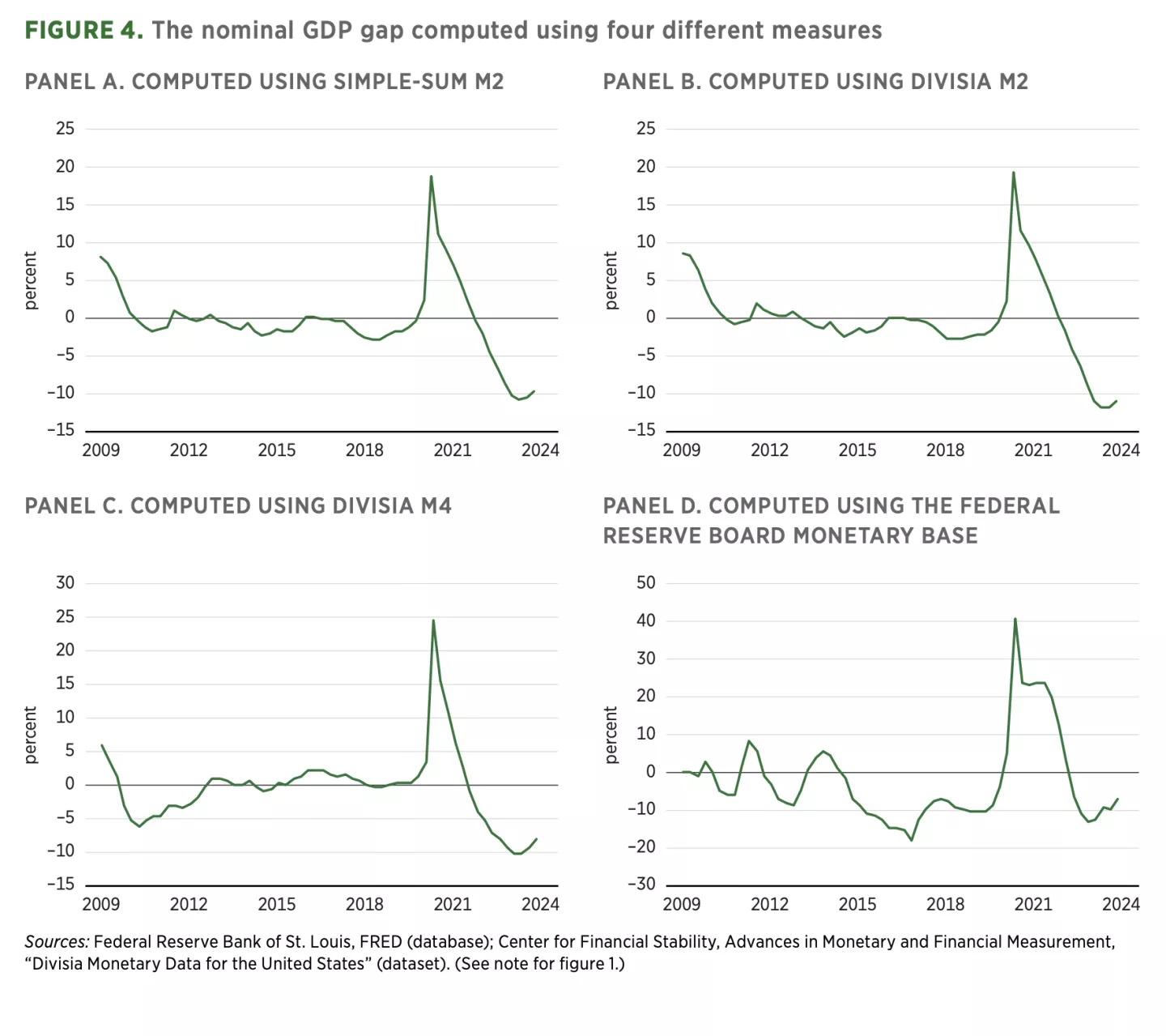
Negative values for all four measures show how monetary policy put downward pressure on NGDP growth from 2011 through 2019. Strongly positive measures show how excess money growth propelled higher NGDP growth and inflation in 2020 and 2021. More recently, however, all four measures have moved back into negative territory. These readings confirm that the interest rate increases implemented by the FOMC in 2022 and 2023 worked as intended, to reduce inflationary pressures. The readings should reassure Fed officials and the general public alike that monetary policy remains consistent with a return of NGDP growth to more normal levels.

About the Author

Peter N. Ireland is a professor of economics at Boston College and a member of the Shadow Open Market Committee.

Acknowledgments

The author would like to thank Patrick Horan and Tracy Miller for very helpful comments on a previous draft of this paper.

Notes

1. This language comes from the 1977 amendment to the Federal Reserve Act, known as the Federal Reserve Reform Act of 1977 (Pub. L. No. 95-188, 91 Stat. 1387, 1387). It appears to give the Fed three objectives: It mentions long-term interest rates as well as employment and prices. As the economist Irving Fisher famously showed, however, lower long-term interest rates follow from lower inflation in the long run. Irving Fisher, “Appreciation and Interest,” Publications of the American Economic Association 11 (July 1896): 1–98. Thus, the act is generally regarded as defining a “dual” mandate for maximum employment and low inflation.

2. For a detailed description of inflation targeting, see Ben S. Bernanke et al., Inflation Targeting: Lessons from the International Experience (Princeton University Press, 1999).

3. Federal Open Market Committee, “Statement on Longer-Run Goals and Monetary Policy Strategy,” January 24, 2012.

4. Federal Open Market Committee, “Statement on Longer-Run Goals and Monetary Policy Strategy,” August 27, 2020.

5. For a detailed analysis of the problems with FAIT, see David Beckworth and Patrick Horan, “The Fate of FAIT: Salvaging the Fed’s Framework” (Mercatus Working Paper, Mercatus Center at George Mason University, October 2022); and Peter N. Ireland, “The Devolution of Federal Reserve Monetary Policy Strategy, 2012–2014” (unpublished manuscript, Boston College, February 2024).

6. Beckworth and Horan, “The Fate of FAIT.”

7. For further discussion of how NGDP targeting improves on FAIT, see David Beckworth, “The Fed’s 2024–25 Framework Review: Optimizing the Dual Mandate Through Nominal GDP Level Targeting” (Mercatus Center at George Mason University, 2024).

8. George Selgin, “Some ‘Serious’ Theoretical Writings That Favor NGDP Targeting,” Cato Institute Blog, June 19, 2018, https://www.cato.org/blog/some-serious-theoretical-writings-favor-ngdp-….

9. Joshua R. Hendrickson, “An Overhaul of Federal Reserve Doctrine: Nominal Income and the Great Moderation,” Journal of Macroeconomics 34 (June 2012): 304–17.

10. Scott Sumner, The Money Illusion: Market Monetarism, the Great Recession, and the Future of Monetary Policy (University of Chicago Press, 2021).

11. David Beckworth, “Facts, Fears, and Functionality of NGDP Level Targeting: A Guide to a Popular Policy Framework for Monetary Policy” (Mercatus Special Study, Mercatus Center at George Mason University, September 2019); Carola Binder, “NGDP Targeting and the Public,” Cato Journal 40 (Spring/Summer 2020): 321–42.

12. Finn E. Kydland and Edward C. Prescott, “Rules Rather Than Discretion: The Inconsistency of Optimal Plans,” Journal of Political Economy 85 (June 1997): 473–92. This article remains the classic reference on the dangers of making monetary policy on the basis of the idea of an exploitable Phillips curve. More recent studies on this point include Robert L. Hetzel, The Federal Reserve: A New History (University of Chicago Press, 2022); Robert L. Hetzel, “What Is the Monetary Standard? The Fed Should Tell Us” (Mercatus Working Paper, Mercatus Center at George Mason University, June 2023); and Ireland, “Devolution of Federal Reserve Monetary Policy Strategy.”

13. Beckworth, “Facts, Fears, and Functionality,” 11–14.

14. David Beckworth and Joshua R. Hendrickson, “Nominal GDP Targeting and the Taylor Rule on an Even Playing Field,” Journal of Money, Credit, and Banking 52 (February 2020): 269–86; Athanasios Orphanides, “Enhancing Resilience with Natural Growth Targeting” (Working Paper 200, Institute for Monetary and Financial Stability at Goethe University, February 2024).

15. John B. Taylor, “Discretion versus Policy Rules in Practice,” Carnegie-Rochester Conference Series on Public Policy 39 (December 1993): 195–214.

16. Scott Sumner, “Using Futures Instrument Prices to Target Nominal Income,” Bulletin of Economic Research 41 (April 1989): 157–62. See also Sumner, The Money Illusion.

17. Bennett T. McCallum, “Robustness Properties of a Rule for Monetary Policy,” Carnegie-Rochester Conference Series on Public Policy 29 (1988): 173–204; Martin Feldstein and James H. Stock, “The Use of a Monetary Aggregate to Target Nominal GDP,” in Monetary Policy, ed. N. Gregory Mankiw (University of Chicago Press, 1994), 7–69.

18. In 2008 the Fed decided to begin paying interest on reserves. The role of this decision in enabling this massive increase in the monetary base to occur without a similar increase in NGDP is discussed in Peter N. Ireland, “Interest on Reserves: History and Rationale, Complications and Risks,” Cato Journal 39 (Spring/Summer 2019): 327–37.

19. The Semiannual Monetary Policy Report to the Congress, Hearing before the Senate Committee on Banking, Housing, and Urban Affairs, 117th Cong. (February 23, 2021) (Jerome H. Powell, Chairman, Board of Governors of the Federal Reserve System).

20. Jeffrey J. Hallman, Richard D. Porter, and David H. Small, “Is the Price Level Tied to the M2 Monetary Aggregate in the Long Run?,” American Economic Review 81 (September 1991): 841–58. For a survey of related research and an application to recent data from several economies, see Peter N. Ireland, “Money Growth and Inflation in the Euro Area, United Kingdom, and United States: Measurement Issues and Recent Results” (unpublished manuscript, Boston College, January 2024).

21. For a full exposition of the quantity-theoretic foundations of the P-star model, see Thomas M. Humphrey, “Precursors of the P-Star Model,” Federal Reserve Bank of Richmond Economic Review 75 (July/August 1989): 3–9.

22. Michael T. Belongia and Peter N. Ireland, “A ‘Working’ Solution to the Question of Nominal GDP Targeting,” Macroeconomic Dynamics 19 (April 2015): 508–34; Michael T. Belongia and Peter N. Ireland, “Circumventing the Zero Lower Bound with Monetary Policy Rules Based on Money,” Journal of Macroeconomics (December 2017): 42–58.

23. For a discussion of the problems monetary policymakers face when estimating the natural rate of output, see Athanasios Orphanides, “Monetary Policy Rules Based on Real-Time Data,” American Economic Review 91 (September 2001): 964–85; and Athanasios Orphanides and Simon van Norden, “The Unreliability of Output-Gap Estimates in Real Time,” Review of Economics and Statistics 84 (November 2002): 569–83.

24. For details on these problems with the original P-star model, see Athanasios Orphanides and Richard D. Porter, “P* Revisited: Money-Based Inflation Forecasts with a Changing Equilibrium Velocity,” Journal of Economics and Business 52 (January–April 2000): 87–100.

25. The original Hodrick-Prescott filter is described in Robert J. Hodrick and Edward C. Prescott, “Postwar U.S. Business Cycles: An Empirical Investigation,” Journal of Money, Credit, and Banking 29 (February 1997): 1–16. The one-sided variant used here is derived by James H. Stock and Mark W. Watson, “Forecasting Inflation,” Journal of Monetary Economics 44 (October 1999): 293–335. Applying the filter in either form requires choosing a value for a parameter λ that governs the relative volatilities of the cyclical and trend components; the setting used here, λ = 1600, is the one recommended for quarterly data by Hodrick and Prescott.

26. The theory of Divisia monetary aggregation was pioneered by William A. Barnett in “Economic Monetary Aggregates: An Application of Index Number and Aggregation Theory,” Journal of Econometrics 14 (September 1980): 11–48. A recent exposition of the theory, together with a review of historical experience favoring the use of Divisia monetary aggregates over their simple-sum counterparts, appears in William A. Barnett, Getting It Wrong: How Faulty Monetary Statistics Undermine the Fed, the Financial System, and the Economy (MIT Press, 2012). The data sources for this paper are listed in the source note for figure 1.

27. When comparing the three panels of figure 1, it is important to note that while simple-sum M2 is measured in units of dollars, Divisia M2 and Divisia M4 are index numbers, normalized to equal 100 in the base year of 1967. Thus, unlike the numerical value of simple-sum M2 velocity, which measures the dollar value of NGDP relative to the dollar value of the money stock, the numerical values of Divisia M2 and Divisia M4 have no special meaning. Instead, the changes in Divisia M2 and Divisia M4 velocities should be compared to those in M2 velocity: All three measure the growth rates of NGDP relative to the corresponding monetary aggregate.

28. John W. Keating et al., “A Model of Monetary Policy Shocks for Financial Crises and Normal Conditions,” Journal of Money, Credit, and Banking 51 (February 2019): 227–59; Cosmas Dery and Apostolos Serletis, “Interest Rates, Money, and Economic Activity,” Macroeconomic Dynamics 25 (October 2021): 1842–91.

29. The St. Louis base series is described by Richard G. Anderson and Robert H. Rasche with Jeffrey Loesel, “A Reconstruction of the Federal Reserve Bank of St. Louis Adjusted Monetary Base and Reserves,” Federal Reserve Bank of St. Louis Review 85 (September/October 2003): 39–69. Deposit sweep programs are discussed by Richard G. Anderson and Robert H. Rasche, “Retail Sweep Programs and Bank Reserves, 1994–1999,” Federal Reserve Bank of St. Louis Review 83 (January/February 2001): 51–72.

30. The 1967 starting date is determined by the availability of data on Divisia M2 and M4 supplied by the Center for Financial Stability.

31. As noted earlier, the St. Louis Fed discontinued its series for the adjusted monetary base, so samples of data using this series must end in quarter 3 of 2019.